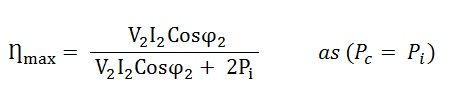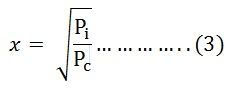AC MACHINES-1 (66761) Theory
6. Understand the efficiency and cooling system of transformer.
6.3. Deduce the equation for maximum efficiency.
How do you calculate the maximum efficiency of a transformer?
Transformer Efficiency
The Efficiency of the transformer is defined as the ratio of useful output power to the input power. The input and output power are measured in the same unit. Its unit is either in Watts (W) or KW. Transformer efficiency is denoted by Ƞ.
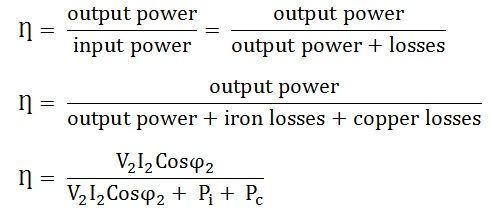
Where,
- V2 – Secondary terminal voltage
- I2 – Full load secondary current
- Cosϕ2 – power factor of the load
- Pi – Iron losses = hysteresis losses + eddy current losses
- Pc – Full load copper losses = I22Res
Consider, the x is the fraction of the full load. The efficiency of the transformer regarding x is expressed as
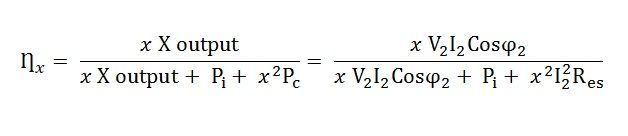
The copper losses vary according to the fraction of the load.
Maximum Efficiency Condition of a Transformer
The efficiency of the transformer along with the load and the power factor is expressed by the given relation:
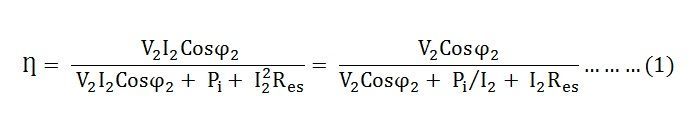 The value of the terminal voltage V2 is approximately constant. Thus, for a given power factor the Transformer efficiency depends upon the load current I2. In equation (1), the numerator is constant and the transformer efficiency will be maximum if the denominator with respect to the variable I2 is equated to zero.
The value of the terminal voltage V2 is approximately constant. Thus, for a given power factor the Transformer efficiency depends upon the load current I2. In equation (1), the numerator is constant and the transformer efficiency will be maximum if the denominator with respect to the variable I2 is equated to zero.
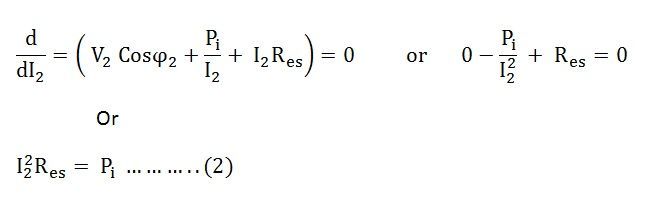 i.e Copper losses = Iron losses
i.e Copper losses = Iron losses
Thus, the transformer will give the maximum efficiency when their copper loss is equal to the iron loss.
From equation (2) the value of output current I2 at which the transformer efficiency will be maximum is given as
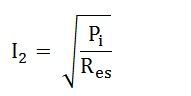
If x is the fraction of full load KVA at which the efficiency of the transformer is maximum then,
Copper losses = x2Pc (where Pc is the full load copper losses)
Iron losses = Pi
For maximum efficiency
x2 Pc = Pi
Therefore
Thus, output KVA corresponding to maximum efficiency
Putting the value of x from the above equation (3) in equation (4) we will get,
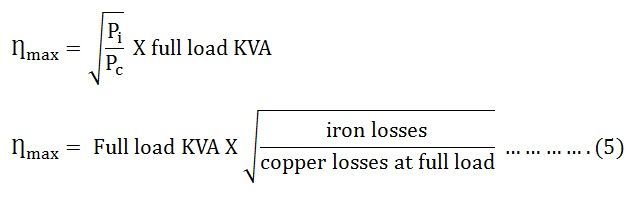 The above equation (5) is the maximum efficiency condition of the transformer.
The above equation (5) is the maximum efficiency condition of the transformer.